– where 2 is the base & 6 is the exponent, and read as “two raised to the power six”
The negative integral exponent of a rational number when a is the nonzero R.N ,
![]() (there is
(there is ![]() and
and ![]() multiplication inverse)
multiplication inverse)
![]()
![]() where m > n
where m > n ![]()
![]()
![]()
Express the numbers in exponential form![]() where
where ![]() e.g=””
e.g=”” ![]() =””
=”” ![]() =”” <strong=””>Square
=”” <strong=””>Square
The square of a number is the product of the number with the number itself
e.g. ![]() ,
, ![]() ,
, ![]()
1,4,9,16,25……… are called perfect squares.</a<10 36 = 2*2*3*3
36 = 2*2*3*3 1^2=1
1^2=1![]() 2^2 =4
2^2 =4![]() n=1∴2n=22^2=4
n=1∴2n=22^2=4![]() 3^2=9
3^2=9![]() n=2 ∴2n=43^2=9
n=2 ∴2n=43^2=9![]() 4^2=16
4^2=16![]() n=3 ∴2n=6
n=3 ∴2n=6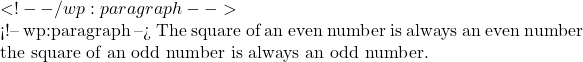 2^2=4
2^2=4![]() 3^2=9
3^2=9![]() 3^2=9=3×34^2=16=3×5+1
3^2=9=3×34^2=16=3×5+1![]() 4^2=16=4×45^2=25=4×6+1$
4^2=16=4×45^2=25=4×6+1$
The square of natural number ending with five follows a delimit pattern
52 = (0×1) hundred + 25 = 25
152 = (1×2) hundred + 25 = 225
252 = (2×3) hundreds + 25 = 625
352 = (3×4) hundred + 25 = 1225
452 = (4×5) hundred + 25 = 2025
The sum of first nodal natural numbers in n2
Sum of first odd number = 1 =12
Sum of first two odd number = 1+3 = 4 = 22
Sum of first three odd number = 1+3+5 = 9 = 32
The sum of rfirst four oddd number = 1+3+5+> = 16 =42
Look at this pattern whose number include only one.
12 = 1 ___________(= 12)
112 = 121 (1+2+1 = 4 = 22)
1112 = 12321 (1+2+3+2+1 = 9 = 32)
11112 = 1234321 (1+2+3+4+3+2+1 = 16 = 42)
111112 = 121 (1+2+3+4+5+4+3+2+1 = 25 = 52)
Square of these numbers is serially overdraft 1,2,3 …………..equal to the number of its digit & decreases vice verse ………3,2,1
The sum of the digit of their product is also a perfect square.
72 = 49 pattern- when number of digit = 1 than number of 4 = n, & number of 8 = n time +1
672 = 4489
6672 = 444889
666722 = 44448889
Pythagorean triplet
In a right-angled triangle:-
For any number m>1, (2m, m2-1, m2 +1) is a Pythagorean triplet
If 3, 4, 5
M=2 22-1, 2×2, 22+1
Square root
The square root of the number is that number, which when multiplied by itself, gives the number as the product.
√x×√x=x we denote the square root of x, by √x, the square root is an inverse process, of the square.
2×2 = 4 & √(4 )= √(2×2) = 2
Note:- if a number has a natural number as a square root then its units digit must be 0,1,4,5,6 or 9. Negative numbers have no square root in the system of natural numbers
e.g √25= ≠5
to find the square root by factorization method
√16= √(4×4)=4
The number being the perfect square, will have one or more pairs of the prime factor, write one factor from each pair & multiplied these factors, the product will be the square root of the number e.g√81= √(3×3×3×3)=3×3=9
To find square root by successive subtraction method
The sum of the first n odd natural numbers is n2
This method is useful to find the square root of smaller natural numbers.
81-1 = 80
80-3 = 77
77-5 = 72
72-7 = 65
65-9 = 56
56-11 = 45
45-13 = 32
32-15 = 17
17-17 = 0
Square root by division method
e.g.
to find the number of digit in the square root
√((81) ̅ ) = 1 digit = 9
√(2 ̅(25) ̅ )=2 digit=25
√((20) ̅(25) ̅ )=2 digit=48
√(2 ̅8 ̅(224) ̅ )=3 digit=168
The square root of rational numbers(fraction)
√(a/b)= √a/√b
The square root of decimal
We see that 0.2 ×0.2 = 0.04 ∴ √(0.(04) ̅ ) = 0.2 number of digit 1 & root of 4 = 2
Approximate value of square root
We get the square of that number multiplied by itself x×x = x2
Similarly, if a number is multiplied by itself 3 times we get the cube of that number x×x×x = x3
Perfect cube:- a natural number is said to be a perfect cube if it is a cube or a natural number.
e.g 13 = 1, 23 = 8, 33 = 27 thus 1,8,27 are perfect cube.
Properties:-
The cube of even numbers are even & odd numbers are odd.
In a perfect cube, each prime number appears three times in its prime factorization √27= √(3×3×3)=
The Cube of negative numbers is negative
Cubes of numbers ending with 0,1,4,5,6 & 9 also end with the same digit ending with 8 will end with Similar cubes of numbers ending in 3 &7 will end with 7 & 3 respectively.
Smallest number:- some numbers are expressed as the same of two squares & sum of two cubes also.
c.g.:-
1729 = 1728 + 1 = 123 +1
1729 = 1000 + 729 = 103 + 93
4104 = 8 + 4096 = 23 + 163, 4104 = 729 + 3375 = 92 + 153
13832 = 5832 + 8000 = 183 + 203, 13832 = 8+13824 = 23 + 243
Cube root of decimal numbers
To find the cube root of aq decimal numbers, write the number in the form of p/q and then find their cube root
Know More
